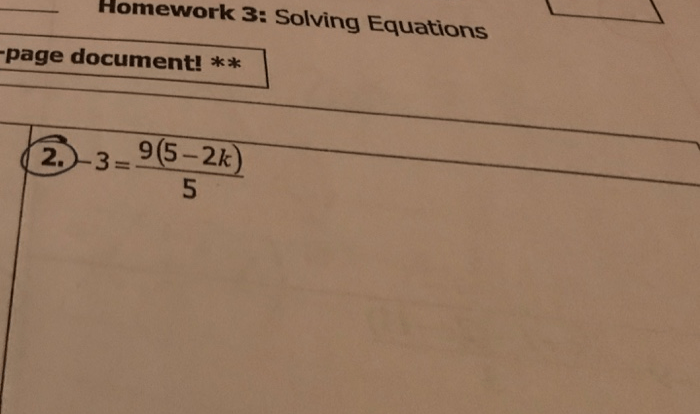
Calculus finney demana waits kennedy – Embarking on a journey through the realm of Calculus: Finney, Demana, Waits, Kennedy, this comprehensive guide unveils the intricate tapestry of mathematical concepts, applications, and pedagogical approaches that define this fundamental discipline.
This authoritative exploration delves into the textbook’s structure, strengths, and weaknesses, while illuminating the practical applications of Calculus in diverse fields, tracing its historical evolution, and examining the transformative impact of technology on its teaching and learning.
Calculus: Finney, Demana, Waits, Kennedy
Calculus: Finney, Demana, Waits, Kennedy is a widely acclaimed textbook for introductory calculus courses. It provides a comprehensive and rigorous treatment of the subject, covering essential concepts, techniques, and applications.
The textbook is organized into three main parts: limits and derivatives, integrals, and applications of calculus. Each part is further divided into chapters that cover specific topics, such as the limit of a function, the derivative of a function, and the integral of a function.
The chapters are well-structured, with clear explanations and numerous examples to illustrate the concepts being discussed.
Strengths of the Textbook
- Clear and concise explanations
- Abundant examples and practice problems
- Comprehensive coverage of topics
- Rigorous mathematical treatment
- Widely adopted by universities and colleges
Weaknesses of the Textbook
- Can be challenging for students with a weak mathematical background
- Some topics are covered in too much detail, which can be overwhelming for some students
- Lacks a strong focus on applications of calculus in real-world scenarios
Applications of Calculus in Real-World Problems
Calculus finds widespread applications in various fields, enabling us to model and solve complex real-world problems. Its fundamental principles and techniques, such as derivatives, integrals, and optimization, provide powerful tools for analyzing and predicting behavior in diverse areas.
Physics
In physics, calculus is essential for describing motion, forces, and energy. Derivatives are used to determine velocity and acceleration, while integrals are employed to calculate displacement, work, and energy. For instance, the trajectory of a projectile can be modeled using calculus, considering factors like initial velocity, angle of projection, and gravitational force.
Engineering
Calculus plays a vital role in engineering disciplines. It is used to analyze structural integrity, optimize designs, and control systems. For example, in civil engineering, calculus is applied to calculate the bending moment and shear forces in bridges and buildings.
In mechanical engineering, it is used to design efficient engines and optimize fluid flow.
Economics
Calculus is widely used in economics to model market behavior, analyze consumer demand, and optimize investment strategies. Derivatives are used to calculate marginal costs and revenues, while integrals are employed to determine total profits and consumer surplus. For instance, calculus can be used to determine the optimal production level for a firm to maximize profits.
Social Sciences
Calculus finds applications in social sciences as well. It is used to model population growth, predict disease spread, and analyze social trends. For example, in epidemiology, calculus is used to develop models for predicting the spread of infectious diseases and evaluating the effectiveness of vaccination programs.
Limitations and Challenges
While calculus is a powerful tool, it also has limitations. Calculus models are simplifications of real-world systems, and they may not always accurately capture all the complexities. Additionally, solving calculus problems can be computationally intensive, especially for complex systems.
Historical Development of Calculus
Calculus, a fundamental branch of mathematics, has its roots in ancient Greece and has undergone significant evolution over centuries. Its origins can be traced back to the work of mathematicians like Eudoxus and Archimedes, who developed methods for calculating areas and volumes of geometric shapes.
The modern foundations of calculus were laid in the 17th century by Isaac Newton and Gottfried Wilhelm Leibniz. Newton, in his work on physics and astronomy, developed a system of calculus based on the concept of fluxions and fluents, while Leibniz independently developed a more systematic approach using infinitesimals.
Contributions of Key Mathematicians, Calculus finney demana waits kennedy
- Isaac Newton (1643-1727):Newton’s work on calculus was primarily motivated by his studies in physics and astronomy. He developed the concept of fluxions and fluents, which allowed him to analyze the rates of change of quantities. Newton’s contributions to calculus include the development of the fundamental theorem of calculus, which establishes the connection between differentiation and integration.
- Gottfried Wilhelm Leibniz (1646-1716):Leibniz independently developed a system of calculus using infinitesimals, which he called the “calculus of infinitesimals.” Leibniz’s notation, which is still widely used today, made calculus more accessible and easier to apply to various problems. He also developed the product and quotient rules of differentiation and the fundamental theorem of calculus.
- Leonhard Euler (1707-1783):Euler made significant contributions to the development of calculus, particularly in the area of differential equations. He introduced the concept of the exponential function and developed the Euler-Mascheroni constant. Euler’s work also laid the foundation for the calculus of variations and the theory of complex functions.
Influence on Scientific and Technological Advancements
The development of calculus has had a profound impact on scientific and technological advancements. It has provided a powerful tool for analyzing and solving problems in various fields, including physics, engineering, economics, and biology.
Calculus has enabled scientists and engineers to make precise predictions about the behavior of the physical world. It has been instrumental in the development of technologies such as calculus of variations, differential equations, and numerical analysis, which have applications in fields such as fluid mechanics, heat transfer, and structural analysis.
Pedagogical Approaches in Calculus Education
Pedagogical approaches in Calculus education vary significantly, with each approach offering unique advantages and disadvantages. Understanding the different approaches can help educators select the most effective methods for teaching Calculus and improving student learning outcomes.
Traditional Lecture-Based Methods
Traditional lecture-based methods involve the instructor presenting information to students in a classroom setting. This approach has been widely used for centuries and remains common in many educational institutions. Key characteristics of traditional lecture-based methods include:
- Instructor-centered delivery of information
- Passive learning by students
- Limited student engagement and interaction
- Emphasis on memorization and recall
Active Learning Methods
Active learning methods emphasize student engagement and participation in the learning process. These methods shift the focus away from the instructor as the sole source of information and towards students as active participants in their own learning. Key characteristics of active learning methods include:
- Student-centered activities
- Hands-on experiences and problem-solving
- Collaborative learning and group work
li>Regular feedback and assessment
Technological Advancements in Calculus: Calculus Finney Demana Waits Kennedy
Technological advancements have had a profound impact on the teaching and learning of Calculus. The advent of graphing calculators, computer algebra systems, and online learning platforms has revolutionized the way students approach and understand the subject.
Graphing calculators have made it possible for students to visualize complex functions and equations, enabling them to gain a deeper understanding of the relationships between variables. They can also be used to perform calculations and solve problems quickly and efficiently, freeing up time for students to focus on conceptual understanding.
Computer Algebra Systems
Computer algebra systems (CAS) are powerful software programs that can perform a wide range of mathematical operations, including symbolic differentiation, integration, and equation solving. CAS can be used to check students’ work, provide step-by-step solutions to problems, and generate graphs and other visualizations.
While CAS can be a valuable tool for learning Calculus, it is important for students to develop a strong understanding of the underlying concepts before relying too heavily on technology.
Online Learning Platforms
Online learning platforms have made it possible for students to access Calculus courses and resources from anywhere in the world. These platforms often provide interactive simulations, video lectures, and discussion forums, which can enhance the learning experience and make Calculus more accessible to a wider range of students.
While technology can be a powerful tool for teaching and learning Calculus, it is important to use it wisely. Technology should be used to supplement traditional methods of instruction, not replace them. Students need to develop a strong foundation in the fundamentals of Calculus before they can effectively use technology to enhance their understanding.
Interdisciplinary Connections of Calculus

Calculus, as a fundamental branch of mathematics, establishes deep connections with various other disciplines, fostering interdisciplinary approaches that enhance our understanding and applications of mathematical concepts.
The interconnectedness of Calculus with other fields allows for a comprehensive and holistic approach to problem-solving and knowledge acquisition.
Algebra
Calculus and Algebra are closely intertwined. Calculus utilizes algebraic operations such as differentiation and integration to analyze and transform algebraic expressions. These operations enable the study of functions, their rates of change, and the areas under their curves.
Geometry
Calculus and Geometry complement each other, providing powerful tools for geometric analysis. Calculus allows for the calculation of volumes, surface areas, and lengths of complex geometric shapes. It also enables the study of curvature and other geometric properties.
Trigonometry
Calculus and Trigonometry are interconnected through the study of periodic functions. Calculus techniques, such as differentiation and integration, are used to analyze trigonometric functions, their derivatives, and integrals. This interplay facilitates the understanding of wave motion and other periodic phenomena.
Physics
Calculus is a cornerstone of Physics, providing essential tools for analyzing motion, forces, and energy. It enables the study of kinematics, dynamics, and thermodynamics through the use of differential equations, integrals, and other calculus concepts.
Questions Often Asked
What is the primary focus of Calculus: Finney, Demana, Waits, Kennedy?
The textbook provides a comprehensive introduction to the fundamental concepts and principles of Calculus, covering topics such as limits, derivatives, integrals, and their applications.
How is Calculus: Finney, Demana, Waits, Kennedy structured?
The textbook is organized into chapters that progress logically, building upon previously introduced concepts. Each chapter includes numerous examples, exercises, and review questions to reinforce understanding.
What are the strengths of Calculus: Finney, Demana, Waits, Kennedy?
The textbook is praised for its clear and concise explanations, abundance of practice problems, and strong emphasis on real-world applications.
What are the weaknesses of Calculus: Finney, Demana, Waits, Kennedy?
Some critics argue that the textbook’s focus on applications may come at the expense of theoretical depth, and that the exercises could be more challenging.